Reflection On The Y Axis

3.3 Graphing Functions Using Reflections about the Axes

Some other transformation that tin be applied to a part is a reflection over the 10– or y-centrality. A vertical reflection reflects a graph vertically across the ten-axis, while a horizontal reflection reflects a graph horizontally across the y-axis. The reflections are shown in Figure 3-9.

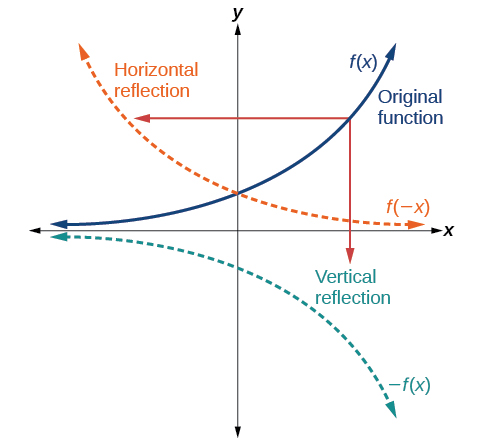
Graph of the vertical and horizontal reflection of a function. — Effigy 3-9: Vertical and horizontal reflections of a function.

Notice that the vertical reflection produces a new graph that is a mirror prototype of the base or original graph about the x-axis. The horizontal reflection produces a new graph that is a mirror prototype of the base or original graph about the y-axis.

Reflections

Given a function [latex]\text{}f\left(10\correct)[/latex], a new role [latex]\text{}chiliad\left(x\right)=-f\left(x\correct)\text{}[/latex] is a vertical reflection of the office [latex]\text{}f\left(x\right)\text{}[/latex], sometimes called a reflection nigh (or over, or through) the 10-axis.

Given a function [latex]\text{}f\left(x\right)\text{}[/latex], a new office [latex]\text{}thousand\left(x\right)=f\left(-x\right)\text{}[/latex] is a horizontal reflection of the function [latex]\text{}f\left(ten\right)\text{}[/latex], sometimes called a reflection virtually the y-axis.

Given a function, reflect the graph both vertically and horizontally.

Multiply all outputs by –i for a vertical reflection. The new graph is a reflection of the original graph about the x-axis.
Multiply all inputs by –one for a horizontal reflection. The new graph is a reflection of the original graph almost the y-centrality.

Reverberate the graph of [latex]\text{}s\left(t\correct)=\sqrt{t}[/latex] (a) vertically and (b) horizontally.
Reflect the graph of [latex]\text{}f\left(x\right)=|x-i|[/latex] (a) vertically and (b) horizontally.

Solution

ane. A function [latex]\text{}f\left(x\right)\text{}[/latex] is given as Tabular array 3. Create a tabular array for the functions below.

[latex]\text{}g\left(10\right)=-f\left(x\right)[/latex]
[latex]\text{}h\left(x\right)=f\left(-ten\correct)[/latex]

Table 3
[latex]x[/latex]	2	four	6	eight
[latex]f\left(x\right)[/latex]	1	3	7	11

ii. A function [latex]\text{}f\left(x\correct)\text{}[/latex] is given as Tabular array 4. Create a table for the functions below.

[latex]one thousand\left(10\right)=-f\left(x\correct)[/latex]
[latex]h\left(x\right)=f\left(-x\right)[/latex]

Tabular array 4
[latex]x[/latex]	−2	0	2	four
[latex]f\left(x\right)[/latex]	v	x	fifteen	twenty

Solution

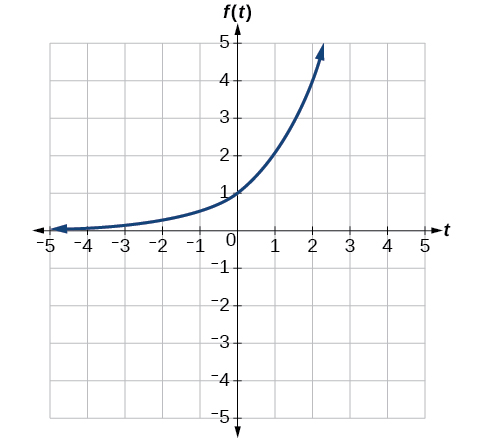
A common model for learning has an equation similar to [latex]k\left(t\right)=-{2}^{-t}+1,\text{}[/latex] where [latex]k[/latex] is the percent of mastery that can be achieved afterward [latex]t[/latex] practice sessions. This is a transformation of the function [latex]f\left(t\right)={2}^{t}[/latex] shown in Figure 3-10. Sketch a graph of [latex]k\left(t\right)[/latex].

Assay

As a model for learning, this function would be express to a domain of [latex]\text{}t\ge 0\text{}[/latex], with corresponding range [latex]\text{}\left[0,1\right)[/latex].

Solution

Given the toolkit function [latex]\text{}f\left(x\correct)={x}^{ii},\text{}[/latex] graph [latex]\text{}m\left(x\correct)=-f\left(x\right)\text{}[/latex] and [latex]\text{}h\left(x\correct)=f\left(-ten\right)\text{}[/latex]. Take note of any surprising behaviour for these functions.

Solution

Access for gratuitous at https://openstax.org/books/precalculus/pages/1-introduction-to-functions